Chargement de l'audio en cours
Plus

Plus

Cours
P.322-326
Mode édition
Ajouter

Ajouter

Terminer

Terminer

Chapitre 12


Cours

1
Deuxième loi de Newton (⇧)


A
Centre de masse d’un système

Le centre de masse d’un système est le point auquel on associe toute la masse du système par simplification. Il exerce autour de lui le même champ de gravitation que le système lui-même.

Pour un solide de masse volumique homogène, le centre de masse correspond au centre géométrique : centre de la sphère, du cube, etc.

B
Référentiels galiléens

Les lois de Newton sont énoncées dans le cadre des référentiels galiléens.

Un référentiel est dit galiléen si le principe d’inertie est vérifié dans celui‑ci. Cela implique qu’un référentiel est galiléen s’il est en mouvement rectiligne uniforme par rapport à un autre référentiel galiléen.

En pratique, si la durée de l’expérience permet de négliger les effets de la rotation ou de l’accélération d’un référentiel, on considérera celui‑ci comme galiléen.

C
Énoncé de la deuxième loi de Newton

Dans un référentiel galiléen, la résultante des forces extérieures ΣF→\Sigma \overrightarrow{F} est égale à :

ΣF→=m ⋅a→\Sigma \overrightarrow{F}=m \ · \overrightarrow{a} ΣF→\Sigma \overrightarrow{F} : résultante des forces extérieures de norme ∥ΣF→∥(N)\|\Sigma \overrightarrow{F}\|(N)
mm : masse du système (kg)
a→\overrightarrow{a} : accélération du système de norme a (m·s-2)

Application : freinage d’une voiture

Représenter les forces s’exerçant sur une voiture lors d’un freinage.

Corrigé :

Le vecteur accélération a la même direction et le même sens que la résultante des forces extérieures.

freinage d’une voiture

Le principe d’inertie, ou première loi de Newton, est un cas particulier de la deuxième loi de Newton, pour lequel la résultante des forces est égale au vecteur nul 0→\overrightarrow{0}.

Un système soumis à une résultante des forces nulle ΣF→=0→\Sigma \overrightarrow{F}=\overrightarrow{0} est nécessairement soit immobile, soit en mouvement rectiligne uniforme, c’est‑à‑dire que a→=0→\overrightarrow{a}=\overrightarrow{0}.

Vocabulaire


Référentiel géocentrique


Référentiel terrestre



Référentiel géocentrique : référentiel dont le centre est le centre de la Terre et dont les trois axes pointent vers trois étoiles supposées fixes.

Référentiel terrestre : également appelé référentiel du laboratoire, il est centré sur un point fixe de la Terre et ses trois axes sont immobiles par rapport à la surface.

Pas de malentendu

➜ Le référentiel terrestre sera considéré comme galiléen pour toutes les expériences de durée faible devant la durée de rotation de la Terre sur elle‑même (24 h).

Doc. 1
Solide à l’équilibre

Solide à l’équilibre

Un système assimilé à un point matériel et soumis à deux forces est à l’équilibre si les deux forces ont la même intensité et des sens opposés.
Ce même système soumis à trois forces est à l’équilibre si :
F1→+F2→+F3→=0→\overrightarrow{F_{1}}+\overrightarrow{F_{2}}+\overrightarrow{F_{3}}=\overrightarrow{0}

Doc. 2
Freinage d’une voiture

Freinage d’une voiture

Éviter les erreurs

➜ La deuxième loi de Newton est une relation vectorielle : lorsque l’on projette cette égalité suivant un axe, il est impératif de tenir compte du sens des vecteurs force considérés.

Supplément numérique

Retrouvez une explication de la deuxième loi de Newton en vidéo :

Matthieu Colombel, Laissemoitaider

Matthieu Colombel, Laissemoitaider

2
Mouvement dans un champ de pesanteur uniforme (⇧)


A
Vecteurs accélération et vitesse

À la surface de la Terre, on considère le champ de pesanteur g→\overrightarrow{g} comme uniforme. Le mouvement du centre de masse G d’un corps de masse mm en chute libre est étudié, dans un référentiel (O,i→,j→,k→)(\text O, \overrightarrow{i}, \overrightarrow{j}, \overrightarrow{k}) (doc. 2).
D’après la deuxième loi de Newton :
ΣF→=m ⋅a→P→=m ⋅a→m ⋅g→=m ⋅a→g→=a→\begin{aligned} \Sigma \overrightarrow{F} &=m \ · \overrightarrow{a} \\ \overrightarrow{P} &=m\ · \overrightarrow{a} \\ m \ · \overrightarrow{g} &=m\ · \overrightarrow{a} \\ \overrightarrow{g} &=\overrightarrow{a} \end{aligned}

Lors d’une chute libre, le vecteur accélération du centre de masse du système a→\overrightarrow{a} est constant et égal au champ de pesanteur g→\overrightarrow{g}.

Les coordonnées de g→\overrightarrow{g} sont donc celles de g→\overrightarrow{g} :
a→=−g ⋅k→\overrightarrow{a}=-g \ · \overrightarrow{k} soit a→(00−g)(O,i→,j→,k→)\overrightarrow{a}\left(\begin{array}{c} 0 \\ 0 \\ -g \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{j}, \overrightarrow{k})}

On peut en déduire les coordonnées du vecteur vitesse v→\overrightarrow{v} par intégration :
v→(v0xv0y−g ⋅t+v0z)(O,i→,j→,k→)\overrightarrow{v}\left(\begin{array}{c} v_{0 x} \\ v_{0 y} \\ -g\ · t+v_{0 z} \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{j}, \overrightarrow{k})}

avec v0xv_{0 x}, v0yv_{0 y} et v0zv_{0 z} les coordonnées du vecteur vitesse initiale v→0\overrightarrow{v}_{0}.

B
Chute sans vitesse initiale

Dans le cas où le solide est lâché sans vitesse initiale, alors v a pour coordonnées :
a→=−g ⋅k→\overrightarrow{a}=-g \ · \overrightarrow{k} soit a→(00−g⋅t)(O,i→,j→,k→)\overrightarrow{a}\left(\begin{array}{c} 0 \\ 0 \\ -g \cdot t \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{j}, \overrightarrow{k})}

Par intégration, on peut donc en déduire la position du centre de masse au cours du temps :
OG→(x0y0−12g ⋅t2+z0)(O,i→,j→,k→)\overrightarrow{\mathrm{OG}}\left(\begin{array}{c} x_{0} \\ y_{0} \\ -\dfrac{1}{2} g \ · t^{2}+z_{0}\\ \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{j}, \overrightarrow{k})}

Si l’origine du repère est placée suivant l’axe de la chute libre, cette équation se simplifie :
OG→=(−12g ⋅t2+z0)⋅k→\overrightarrow{\mathrm{OG}}=\left(-\dfrac{1}{2} g\ · t^{2}+z_{0}\right) · \overrightarrow{k}

Lors d’une chute libre sans vitesse initiale, la vitesse est proportionnelle à la durée de la chute et la trajectoire est verticale.

C
Mouvement parabolique

Lors d’une chute dans un champ de pesanteur uniforme, le mouvement est plan et peut être étudié dans le seul repère (O,i→,k→)(\text O, \overrightarrow{i}, \overrightarrow{k}).

Dans le cas où le solide est lancé avec une vitesse initiale formant un angle α\alpha avec l’horizontal, le vecteur v→0\overrightarrow{v}_{0} a pour composantes :
v→0(v0⋅cos⁡(α)v0⋅sin⁡(α))(O,i→,k→)\overrightarrow{v}_{0}\left(\begin{array}{c} v_{0} · \cos (\alpha) \\ v_{0} · \sin (\alpha) \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{k})}

Cela implique que :
v→(v0⋅cos⁡(α)−g ⋅t+v0⋅sin⁡(α))(O,i→,k→)\overrightarrow{v}\left(\begin{array}{l} v_{0} · \cos (\alpha) \\ -g \ · t+v_{0} · \sin (\alpha) \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{k})}

Par intégration :
OG→(x(t)=v0 ⋅cos⁡(α) ⋅t+x0z(t)=−12g ⋅t2+v0 ⋅sin⁡(α) ⋅t+z0)(O,i→,k→)\overrightarrow{\mathrm{OG}}\left(\begin{array}{l} x(t)=v_{0}\ · \cos (\alpha) \ · t+x_{0} \\ z(t)=-\dfrac{1}{2} g\ · t^{2}+v_{0} \ · \sin (\alpha)\ · t+z_{0} \end{array}\right)_{(\text O, \overrightarrow{i}, \overrightarrow{k})}

L’équation de la trajectoire s’obtient en exprimant zz en fonction de xx. Si la position initiale correspond avec l’origine du repère x0=y0=z0=0x_{0}=y_{0}=z_{0}=0 m, on a alors t=xv0⋅cos⁡(α)t=\dfrac{x}{v_{0} · \cos (\alpha)} d'où :
z(x)=−12g ⋅x2v02 ⋅cos⁡(α)2+tan⁡(α) ⋅xz(x)=-\dfrac{1}{2} g \ · \dfrac{x^{2}}{v_{0}^{2}}\ · \cos (\alpha)^{2}+\tan (\alpha) \ · x

Si la vitesse initiale est non nulle et non verticale, la trajectoire est parabolique.

D
Exploitation des équations

Les équations horaires et de la trajectoire permettent de déterminer différentes grandeurs caractéristiques du mouvement : flèche λλ, portée DD, angle de tir α\alpha, etc.

Application : expression de la flèche d’un mouvement parabolique

La flèche, notée HH, correspond à l’altitude du sommet S de la parabole. En ce point, la vitesse est horizontale, donc :
−g ⋅ts+v0 ⋅sin⁡(α)=0-g \ · t_{s}+v_{0} \ · \sin (\alpha)=0
ts=v0 ⋅sin⁡(α)gt_{s}=\dfrac{v_{0} \ · \sin (\alpha)}{g}

En remplaçant dans l’équation horaire de z(t)z(t), on obtient :
H=zsH=−12g ⋅ts2+v0⋅sin⁡(α) ⋅tsH=−12g ⋅(v0⋅sin⁡(α)g)2+(v0⋅sin⁡(α))2gH=(v0⋅sin⁡(α))22g\begin{array}{l} H=z_{s} \\ H=-\dfrac{1}{2} g\ · t_{s}^{2}+v_{0} · \sin (\alpha) \ · t_{s} \\ H=-\dfrac{1}{2} g\ ·\left(\dfrac{v_{0} · \sin (\alpha)}{g}\right)^{2}+\dfrac{\left(v_{0} · \sin (\alpha)\right)^{2}}{g} \\ H=\dfrac{\left(v_{0} · \sin (\alpha)\right)^{2}}{2 g} \end{array}

Vocabulaire

Champ uniforme


Chute libre



Champ uniforme : un champ est uniforme s’il a même direction, même sens et même intensité en tout point de l’espace.

Chute libre : un corps est en chute libre s’il n’est soumis qu’à son poids.

Doc. 3
Corps en chute libre

Corps en chute libre

Éviter les erreurs

➜ Attention à bien différencier les notations : v→\overrightarrow{v} désigne le vecteur vitesse à un instant tt quelconque, v→0\overrightarrow{v}_{0} désigne le vecteur vitesse initiale.

➜ Ne pas oublier les constantes en écrivant les primitives des coordonnées des vecteurs.

Pas de malentendu

➜ Il ne faut pas confondre les coordonnées d’un vecteur et sa norme.

➜ L’intensité de pesanteur est également appelée accélération de pesanteur. Cette grandeur s’exprime couramment en (N·kg-1), mais correspond à des (m·s‑2) dans le Système international.

Doc. 4
Saut en chute libre

Saut en chute libre

Doc. 5
Conditions initiales

Conditions initiales

Pas de malentendu

➜ Aucune équation horaire ou de trajectoire n’est à retenir : il faut savoir les établir.

➜ Les notations de l’énoncé et la direction des axes du repère peuvent varier : il faut s’adapter.

Doc. 6
Chute libre parabolique

Chute libre parabolique

Doc. 7
Mouvement parabolique

Mouvement parabolique

3
Mouvement dans un champ électrique uniforme (⇧)


A
Champ électrique dans un condensateur plan

Un condensateur plan est constitué de deux armatures métalliques planes parallèles, séparées par un matériau isolant.

Il règne un champ électrique E→\overrightarrow{E}, uniforme et égal à :
E→=−Ud⋅j→\overrightarrow{E}=-\dfrac{U}{d} · \overrightarrow{j}
E→\overrightarrow{E} : champ électrique de norme E (V·m-1)
UU : tension entre les armatures (V)
dd : distance entre les armatures (m)
j→\overrightarrow{j} : vecteur unitaire dirigé de la plaque négative vers la plaque positive

Application

Déterminer l’équation de trajectoire d’une particule de charge qq et de masse mm dans un champ électrique uniforme.

Corrigé :

Une particule de charge qq et de masse mm se trouvant dans ce champ électrique est soumise à la force électrique F→e=q ⋅E→\overrightarrow{F}_{\mathrm{e}}=q\ · \overrightarrow{E}. Le poids de la particule est négligeable devant la force électrique. D’après la deuxième loi de Newton appliquée à la particule :
F→e=m ⋅a→\overrightarrow{F}_{\mathrm{e}}=m \ · \overrightarrow{a}
a→=q ⋅E→m\overrightarrow{a}=\dfrac{q \ · \overrightarrow{E}}{m}

D’après le doc. 8, dans le repère (O,i→,j→)(\mathrm{O}, \overrightarrow{i}, \overrightarrow{j}), on considère une particule, notée M, pénétrant en O à la vitesse v→0\overrightarrow{v}_{0} horizontale :
a→(0−q ⋅Em)(O,i→,j→)\overrightarrow{a}\left(\begin{array}{c} 0 \\ -\dfrac{q \ · E}{m} \end{array}\right)_{(\mathrm{O}, \overrightarrow{i}, \overrightarrow{j})}

Par intégrations successives, on obtient :
v→(v0−q ⋅E→m⋅t)(O,i→,j→)\overrightarrow{v}\left(\begin{array}{c} v_{0} \\ -\dfrac{q \ · \overrightarrow E}{m} · t \end{array}\right)_{(\mathrm{O}, \overrightarrow{i}, \overrightarrow{j})} et OM→(x(t)=v0 ⋅ty(t)=−q ⋅E2m⋅t2)(O,i→,j→)\overrightarrow{\mathrm{OM}}\left(\begin{array}{l} x(t)=v_{0}\ · t \\ y(t)=-\dfrac{q \ · E}{2 m} · t^{2} \end{array}\right)_{(\mathrm{O}, \overrightarrow{i}, \overrightarrow{j})}

D’où l’équation de la trajectoire :
y=−q ⋅E2m ⋅v02⋅x2y=\dfrac{-q \ · E}{2 m\ · v_{0}^{2}} · x^{2}

Application

La particule est un électron. Déterminer le rapport em\dfrac{e}{m}.

Corrigé :

L’équation de la trajectoire devient :
ys=e ⋅E2me⋅v02⋅L2eme=2v02 ⋅ysE ⋅L2AN:eme=2×(2,27×107)2×1,85×10−215,0×103×(8,50×10−2)2=1,76×1011C⋅kg−1\begin{aligned} y_{\mathrm{s}} &=\dfrac{e \ · E}{2 m_{\mathrm{e}} · v_{0}^{2}} · L^{2} \\ \dfrac{e}{m_{\mathrm{e}}} &=\dfrac{2 v_{0}^{2} \ · y_{\mathrm{s}}}{E \ · L^{2}} \\ \mathrm{AN}: \dfrac{e}{m_{\mathrm{e}}} &=\dfrac{2 \times\left(2,27 \times 10^{7}\right)^{2} \times 1,85 \times 10^{-2}}{15,0 \times 10^{3} \times\left(8,50 \times 10^{-2}\right)^{2}}=1,76 \times 10^{11} \mathrm{C} · \mathrm{kg}^{-1} \end{aligned}

B
Principe d’un accélérateur linéaire

Principe d’un accélérateur linéaire

Un accélérateur linéaire de particules est un dispositif permettant de communiquer de l’énergie à des particules chargées. Il est constitué :
    • d’une source de particules (ions, électrons, etc.) ;
    • d’un ensemble de tubes sous vide de longueur croissante, séparés par des interstices entre lesquels règne un champ électrique ;
    • éventuellement d’une cible ou d’un détecteur.

Un générateur de tension alternative permet de faire varier les signes des électrodes et de changer le sens des champs électriques.

Une particule est toujours attirée par une section de tube de signe contraire à sa propre charge.

Doc. 8
Particule dans un champ électrique

Particule dans un champ électrique

Éviter les erreurs

➜ Le sens de déviation (vers l’une ou l’autre des plaques) dépend du signe de la charge de la particule.

➜ S’assurer de la cohérence des résultats en vérifiant qu’une particule chargée positivement est bien attirée par la plaque négative… et vice versa !

Données

  • Vitesse initiale de l’électron : v0=2,27×107v_{0}=2,27 \times 10^{7} m·s-1
  • Intensité du champ électrique : E=15,0E=15,0 kV·m‑1
  • Distance entre les plaques : L=8,50L = 8,50 cm
  • Ordonnée du point S : yS=1,85y_{\mathrm{S}}=1,85 cm

Vocabulaire


Accélérateur linéaire



Accélérateur linéaire : dispositif permettant d’accélérer des particules chargées dans le but de produire des réactions avec la matière. Il est souvent désigné par son acronyme LINAC (LINear ACcelerator).

Doc. 9
Radiothérapie

Radiothérapie

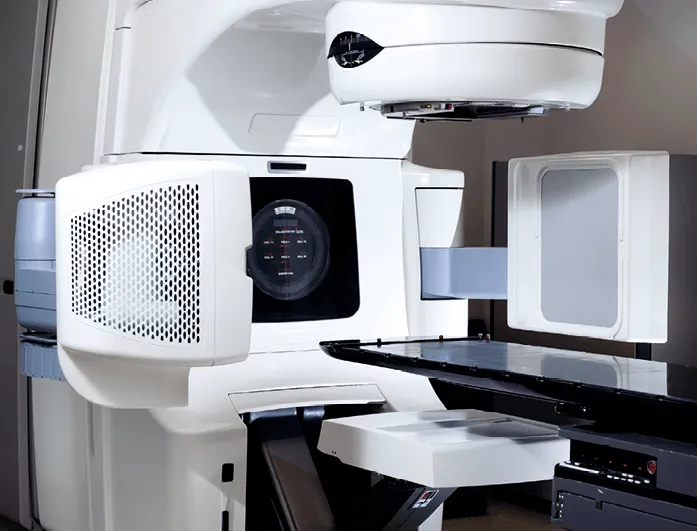
► La radiothérapie est une technique médicale utilisant un accélérateur de particules.

Éviter les erreurs

➜ L’énergie potentielle de pesanteur EppE_{\mathrm{pp}} dépend d’une altitude de référence choisie.

➜ Epp=m ⋅g ⋅zE_{\mathrm{pp}}=m \ · g \ · z si la valeur nulle de EppE_{\mathrm{pp}} est choisie au niveau du sol.

4
Aspects énergétiques (⇧)


A
Énergie mécanique

Pour rappel, l’énergie mécanique EmE_{m} d’un système est définie comme la somme des énergies cinétique et potentielle. Elle se conserve lorsque le système n’est soumis qu’à des forces conservatives comme le poids P→\overrightarrow{P} ou la force électrique Fe→\overrightarrow{F_e}. Lorsque l’énergie mécanique ne se conserve pas, sa variation ΔEm(A→B)\Delta E_{m}(\mathrm{A} \rightarrow \mathrm{B}), pour un système se déplaçant d’un point A\text{A} à un point B\text{B}, est égale à :
ΔEm(A→B)=Em(B)−Em(A)=ΣWAB(Fnc→)\Delta E_{m}(\mathrm{A} \rightarrow \mathrm{B})=E_{m}(\mathrm{B})-E_{m}(\mathrm{A})=\Sigma W_{\mathrm{A B}}(\overrightarrow{F_{\mathrm{n c}}})

ΔEm(A→B)\Delta E_{m}(\mathrm{A} \rightarrow \mathrm{B}) : variation d’énergie mécanique entre A et B (J)
WAB(Fnc→)W_{\mathrm{A B}}(\overrightarrow{F_{\mathrm{n c}}}) : somme des travaux des forces non conservatives (J)

B
Théorème de l’énergie cinétique

Dans un référentiel galiléen, la variation de l’énergie cinétique d’un système de masse mm est égale à la somme des travaux des forces s’exerçant sur le système entre les points A\text{A} et B\text{B} :
ΔEc(A→B)=12m ⋅vB2−12m ⋅vA2=ΣWAB(F→)\Delta E_{c}(\mathrm{A} \rightarrow \mathrm{B})=\dfrac{1}{2} m\ · v_{\mathrm{B}}^{2}-\dfrac{1}{2} m\ · v_{\mathrm{A}}^{2}=\Sigma W_{\mathrm{A B}}(\overrightarrow{F})


Dans le cas d’un mouvement dans le champ de pesanteur, la seule force étant le poids P→\overrightarrow{P} :

ΔEc(A→B)=m ⋅g ⋅(zA−zB)\Delta E_{\mathrm{c}}(\mathrm{A} \rightarrow \mathrm{B})=m \ · g \ ·\left(z_{\mathrm{A}}-z_{\mathrm{B}}\right)

Dans le cas d’une particule accélérée par un champ électrique E→\overrightarrow E , la seule force s’exerçant étant la force électrique :

ΔEc(A→B)=q⋅UAB\Delta E_{c}(\text A \rightarrow \text B)=q \cdot U_\text{AB}

Doc. 10
Fusée au décollage

Fusée au décollage

L’énergie mécanique d’une fusée qui décolle n’est pas constante, car la force de poussée exercée par ses moteurs n’est pas conservative et sa masse n’est pas constante.

Pas de malentendu

➜ Afin de résoudre un exercice, on peut utiliser indifféremment le théorème de l’énergie cinétique ou celui de la conservation de l’énergie mécanique.

Doc. 11
Travail d’une force électrique

Travail d’une force électrique
Le travail de la force électrique est égal à :
W(Fe→)=Fe→⋅AB→=q ⋅UABW(\overrightarrow{F_{\mathrm{e}}})=\overrightarrow{F_{\mathrm{e}}} · \overrightarrow{\mathrm{AB}}=q \ · U_{\mathrm{AB}}
Utilisation des cookies
En poursuivant votre navigation sans modifier vos paramètres, vous acceptez l'utilisation des cookies permettant le bon fonctionnement du service.
Pour plus d’informations, cliquez ici.